|
Nektar++
|
|
Nektar++
|
#include <PrismExp.h>
Public Member Functions | |
| PrismExp (const LibUtilities::BasisKey &Ba, const LibUtilities::BasisKey &Bb, const LibUtilities::BasisKey &Bc, const SpatialDomains::PrismGeomSharedPtr &geom) | |
| Constructor using BasisKey class for quadrature points and order definition. | |
| PrismExp (const PrismExp &T) | |
| ~PrismExp () | |
 Public Member Functions inherited from Nektar::StdRegions::StdPrismExp Public Member Functions inherited from Nektar::StdRegions::StdPrismExp | |
| StdPrismExp () | |
| StdPrismExp (const LibUtilities::BasisKey &Ba, const LibUtilities::BasisKey &Bb, const LibUtilities::BasisKey &Bc) | |
| StdPrismExp (const LibUtilities::BasisKey &Ba, const LibUtilities::BasisKey &Bb, const LibUtilities::BasisKey &Bc, NekDouble *coeffs, NekDouble *phys) | |
| StdPrismExp (const StdPrismExp &T) | |
| ~StdPrismExp () | |
 Public Member Functions inherited from Nektar::StdRegions::StdExpansion3D Public Member Functions inherited from Nektar::StdRegions::StdExpansion3D | |
| StdExpansion3D () | |
| StdExpansion3D (int numcoeffs, const LibUtilities::BasisKey &Ba, const LibUtilities::BasisKey &Bb, const LibUtilities::BasisKey &Bc) | |
| StdExpansion3D (const StdExpansion3D &T) | |
| virtual | ~StdExpansion3D () |
| void | PhysTensorDeriv (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray_d1, Array< OneD, NekDouble > &outarray_d2, Array< OneD, NekDouble > &outarray_d3) |
| Calculate the 3D derivative in the local tensor/collapsed coordinate at the physical points. | |
| void | BwdTrans_SumFacKernel (const Array< OneD, const NekDouble > &base0, const Array< OneD, const NekDouble > &base1, const Array< OneD, const NekDouble > &base2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp, bool doCheckCollDir0, bool doCheckCollDir1, bool doCheckCollDir2) |
| void | IProductWRTBase_SumFacKernel (const Array< OneD, const NekDouble > &base0, const Array< OneD, const NekDouble > &base1, const Array< OneD, const NekDouble > &base2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp, bool doCheckCollDir0, bool doCheckCollDir1, bool doCheckCollDir2) |
 Public Member Functions inherited from Nektar::StdRegions::StdExpansion Public Member Functions inherited from Nektar::StdRegions::StdExpansion | |
| StdExpansion () | |
| Default Constructor. | |
| StdExpansion (const int numcoeffs, const int numbases, const LibUtilities::BasisKey &Ba=LibUtilities::NullBasisKey, const LibUtilities::BasisKey &Bb=LibUtilities::NullBasisKey, const LibUtilities::BasisKey &Bc=LibUtilities::NullBasisKey) | |
| Constructor. | |
| StdExpansion (const StdExpansion &T) | |
| Copy Constructor. | |
| virtual | ~StdExpansion () |
| Destructor. | |
| int | GetNumBases () const |
| This function returns the number of 1D bases used in the expansion. | |
| const Array< OneD, const LibUtilities::BasisSharedPtr > & | GetBase () const |
| This function gets the shared point to basis. | |
| const LibUtilities::BasisSharedPtr & | GetBasis (int dir) const |
| This function gets the shared point to basis in the dir direction. | |
| int | GetNcoeffs (void) const |
| This function returns the total number of coefficients used in the expansion. | |
| int | GetTotPoints () const |
| This function returns the total number of quadrature points used in the element. | |
| LibUtilities::BasisType | GetBasisType (const int dir) const |
| This function returns the type of basis used in the dir direction. | |
| int | GetBasisNumModes (const int dir) const |
| This function returns the number of expansion modes in the dir direction. | |
| int | EvalBasisNumModesMax (void) const |
| This function returns the maximum number of expansion modes over all local directions. | |
| LibUtilities::PointsType | GetPointsType (const int dir) const |
| This function returns the type of quadrature points used in the dir direction. | |
| int | GetNumPoints (const int dir) const |
| This function returns the number of quadrature points in the dir direction. | |
| const Array< OneD, const NekDouble > & | GetPoints (const int dir) const |
| This function returns a pointer to the array containing the quadrature points in dir direction. | |
| int | GetNverts () const |
| This function returns the number of vertices of the expansion domain. | |
| int | GetNedges () const |
| This function returns the number of edges of the expansion domain. | |
| int | GetEdgeNcoeffs (const int i) const |
| This function returns the number of expansion coefficients belonging to the i-th edge. | |
| int | GetTotalEdgeIntNcoeffs () const |
| int | GetEdgeNumPoints (const int i) const |
| This function returns the number of quadrature points belonging to the i-th edge. | |
| int | DetCartesianDirOfEdge (const int edge) |
| const LibUtilities::BasisKey | DetEdgeBasisKey (const int i) const |
| const LibUtilities::BasisKey | DetFaceBasisKey (const int i, const int k) const |
| int | GetFaceNumPoints (const int i) const |
| This function returns the number of quadrature points belonging to the i-th face. | |
| int | GetFaceNcoeffs (const int i) const |
| This function returns the number of expansion coefficients belonging to the i-th face. | |
| int | GetFaceIntNcoeffs (const int i) const |
| int | GetTotalFaceIntNcoeffs () const |
| LibUtilities::PointsKey | GetFacePointsKey (const int i, const int j) const |
| int | NumBndryCoeffs (void) const |
| int | NumDGBndryCoeffs (void) const |
| LibUtilities::BasisType | GetEdgeBasisType (const int i) const |
| This function returns the type of expansion basis on the i-th edge. | |
| int | GetNfaces () const |
| This function returns the number of faces of the expansion domain. | |
| int | GetNtrace () const |
| Returns the number of trace elements connected to this element. | |
| LibUtilities::ShapeType | DetShapeType () const |
| This function returns the shape of the expansion domain. | |
| int | GetShapeDimension () const |
| bool | IsBoundaryInteriorExpansion () |
| void | BwdTrans (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| This function performs the Backward transformation from coefficient space to physical space. | |
| void | FwdTrans (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| This function performs the Forward transformation from physical space to coefficient space. | |
| void | FwdTrans_BndConstrained (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| NekDouble | Integral (const Array< OneD, const NekDouble > &inarray) |
| This function integrates the specified function over the domain. | |
| void | FillMode (const int mode, Array< OneD, NekDouble > &outarray) |
| This function fills the array outarray with the mode-th mode of the expansion. | |
| void | IProductWRTBase (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| this function calculates the inner product of a given function f with the different modes of the expansion | |
| void | IProductWRTBase (const Array< OneD, const NekDouble > &base, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, int coll_check) |
| void | IProductWRTDerivBase (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| int | GetElmtId () |
| Get the element id of this expansion when used in a list by returning value of m_elmt_id. | |
| void | SetElmtId (const int id) |
| Set the element id of this expansion when used in a list by returning value of m_elmt_id. | |
| void | GetCoords (Array< OneD, NekDouble > &coords_1, Array< OneD, NekDouble > &coords_2=NullNekDouble1DArray, Array< OneD, NekDouble > &coords_3=NullNekDouble1DArray) |
| this function returns the physical coordinates of the quadrature points of the expansion | |
| void | GetCoord (const Array< OneD, const NekDouble > &Lcoord, Array< OneD, NekDouble > &coord) |
| given the coordinates of a point of the element in the local collapsed coordinate system, this function calculates the physical coordinates of the point | |
| DNekMatSharedPtr | GetStdMatrix (const StdMatrixKey &mkey) |
| DNekBlkMatSharedPtr | GetStdStaticCondMatrix (const StdMatrixKey &mkey) |
| IndexMapValuesSharedPtr | GetIndexMap (const IndexMapKey &ikey) |
| const Array< OneD, const NekDouble > & | GetPhysNormals (void) |
| void | SetPhysNormals (Array< OneD, const NekDouble > &normal) |
| virtual void | SetUpPhysNormals (const int edge) |
| void | NormVectorIProductWRTBase (const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, Array< OneD, NekDouble > &outarray) |
| void | NormVectorIProductWRTBase (const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, const Array< OneD, const NekDouble > &Fz, Array< OneD, NekDouble > &outarray) |
| DNekScalBlkMatSharedPtr | GetLocStaticCondMatrix (const LocalRegions::MatrixKey &mkey) |
| void | DropLocStaticCondMatrix (const LocalRegions::MatrixKey &mkey) |
| StdRegions::Orientation | GetForient (int face) |
| StdRegions::Orientation | GetEorient (int edge) |
| StdRegions::Orientation | GetPorient (int point) |
| StdRegions::Orientation | GetCartesianEorient (int edge) |
| void | SetCoeffsToOrientation (Array< OneD, NekDouble > &coeffs, StdRegions::Orientation dir) |
| void | SetCoeffsToOrientation (StdRegions::Orientation dir, Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| int | CalcNumberOfCoefficients (const std::vector< unsigned int > &nummodes, int &modes_offset) |
| void | ExtractDataToCoeffs (const NekDouble *data, const std::vector< unsigned int > &nummodes, const int nmodes_offset, NekDouble *coeffs) |
| NekDouble | StdPhysEvaluate (const Array< OneD, const NekDouble > &Lcoord, const Array< OneD, const NekDouble > &physvals) |
| int | GetCoordim () |
| void | GetBoundaryMap (Array< OneD, unsigned int > &outarray) |
| void | GetInteriorMap (Array< OneD, unsigned int > &outarray) |
| int | GetVertexMap (const int localVertexId, bool useCoeffPacking=false) |
| void | GetEdgeInteriorMap (const int eid, const Orientation edgeOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray) |
| void | GetFaceInteriorMap (const int fid, const Orientation faceOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray) |
| void | GetEdgeToElementMap (const int eid, const Orientation edgeOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray) |
| void | GetFaceToElementMap (const int fid, const Orientation faceOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray, int nummodesA=-1, int nummodesB=-1) |
| void | GetEdgePhysVals (const int edge, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| Extract the physical values along edge edge from inarray into outarray following the local edge orientation and point distribution defined by defined in EdgeExp. | |
| void | GetEdgePhysVals (const int edge, const boost::shared_ptr< StdExpansion > &EdgeExp, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | GetTracePhysVals (const int edge, const boost::shared_ptr< StdExpansion > &EdgeExp, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | GetVertexPhysVals (const int vertex, const Array< OneD, const NekDouble > &inarray, NekDouble &outarray) |
| void | GetEdgeInterpVals (const int edge, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | GetEdgeQFactors (const int edge, Array< OneD, NekDouble > &outarray) |
| Extract the metric factors to compute the contravariant fluxes along edge edge and stores them into outarray following the local edge orientation (i.e. anticlockwise convention). | |
| void | GetFacePhysVals (const int face, const boost::shared_ptr< StdExpansion > &FaceExp, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, StdRegions::Orientation orient=eNoOrientation) |
| void | MultiplyByQuadratureMetric (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | MultiplyByStdQuadratureMetric (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| DNekMatSharedPtr | CreateGeneralMatrix (const StdMatrixKey &mkey) |
this function generates the mass matrix ![$\mathbf{M}[i][j] = \int \phi_i(\mathbf{x}) \phi_j(\mathbf{x}) d\mathbf{x}$](form_123.png) | |
| void | GeneralMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | MassMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LaplacianMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | ReduceOrderCoeffs (int numMin, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | SVVLaplacianFilter (Array< OneD, NekDouble > &array, const StdMatrixKey &mkey) |
| void | LaplacianMatrixOp (const int k1, const int k2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | WeakDerivMatrixOp (const int i, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | WeakDirectionalDerivMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | MassLevelCurvatureMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LinearAdvectionDiffusionReactionMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey, bool addDiffusionTerm=true) |
| void | HelmholtzMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| DNekMatSharedPtr | GenMatrix (const StdMatrixKey &mkey) |
| void | PhysDeriv (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_d0, Array< OneD, NekDouble > &out_d1=NullNekDouble1DArray, Array< OneD, NekDouble > &out_d2=NullNekDouble1DArray) |
| void | PhysDeriv (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | PhysDeriv_s (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_ds) |
| void | PhysDeriv_n (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_dn) |
| void | PhysDirectionalDeriv (const Array< OneD, const NekDouble > &inarray, const Array< OneD, const NekDouble > &direction, Array< OneD, NekDouble > &outarray) |
| void | StdPhysDeriv (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_d0, Array< OneD, NekDouble > &out_d1=NullNekDouble1DArray, Array< OneD, NekDouble > &out_d2=NullNekDouble1DArray) |
| void | StdPhysDeriv (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | AddRobinMassMatrix (const int edgeid, const Array< OneD, const NekDouble > &primCoeffs, DNekMatSharedPtr &inoutmat) |
| void | AddRobinEdgeContribution (const int edgeid, const Array< OneD, const NekDouble > &primCoeffs, Array< OneD, NekDouble > &coeffs) |
| NekDouble | PhysEvaluate (const Array< OneD, const NekDouble > &coords, const Array< OneD, const NekDouble > &physvals) |
| This function evaluates the expansion at a single (arbitrary) point of the domain. | |
| NekDouble | PhysEvaluate (const Array< OneD, DNekMatSharedPtr > &I, const Array< OneD, const NekDouble > &physvals) |
| This function evaluates the expansion at a single (arbitrary) point of the domain. | |
| void | LocCoordToLocCollapsed (const Array< OneD, const NekDouble > &xi, Array< OneD, NekDouble > &eta) |
| Convert local cartesian coordinate xi into local collapsed coordinates eta. | |
| const boost::shared_ptr < SpatialDomains::GeomFactors > & | GetMetricInfo (void) const |
| virtual int | v_GetElmtId () |
| Get the element id of this expansion when used in a list by returning value of m_elmt_id. | |
| virtual const Array< OneD, const NekDouble > & | v_GetPhysNormals (void) |
| virtual void | v_SetPhysNormals (Array< OneD, const NekDouble > &normal) |
| virtual void | v_SetUpPhysNormals (const int edge) |
| virtual void | v_NormVectorIProductWRTBase (const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, Array< OneD, NekDouble > &outarray) |
| virtual void | v_NormVectorIProductWRTBase (const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, const Array< OneD, const NekDouble > &Fz, Array< OneD, NekDouble > &outarray) |
| virtual StdRegions::Orientation | v_GetEorient (int edge) |
| virtual StdRegions::Orientation | v_GetCartesianEorient (int edge) |
| virtual StdRegions::Orientation | v_GetPorient (int point) |
| NekDouble | Linf (const Array< OneD, const NekDouble > &phys, const Array< OneD, const NekDouble > &sol=NullNekDouble1DArray) |
Function to evaluate the discrete 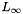 error error 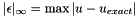 where where  is given by the array sol. is given by the array sol. | |
| NekDouble | L2 (const Array< OneD, const NekDouble > &phys, const Array< OneD, const NekDouble > &sol=NullNekDouble1DArray) |
Function to evaluate the discrete 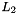 error, error, ![$ | \epsilon |_{2} = \left [ \int^1_{-1} [u - u_{exact}]^2 dx \right]^{1/2} d\xi_1 $](form_135.png) where where  is given by the array sol. is given by the array sol. | |
| NekDouble | H1 (const Array< OneD, const NekDouble > &phys, const Array< OneD, const NekDouble > &sol=NullNekDouble1DArray) |
Function to evaluate the discrete 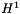 error, error, ![$ | \epsilon |^1_{2} = \left [ \int^1_{-1} [u - u_{exact}]^2 + \nabla(u - u_{exact})\cdot\nabla(u - u_{exact})\cdot dx \right]^{1/2} d\xi_1 $](form_138.png) where where  is given by the array sol. is given by the array sol. | |
| const NormalVector & | GetEdgeNormal (const int edge) const |
| void | ComputeEdgeNormal (const int edge) |
| void | NegateEdgeNormal (const int edge) |
| bool | EdgeNormalNegated (const int edge) |
| void | ComputeFaceNormal (const int face) |
| void | NegateFaceNormal (const int face) |
| void | ComputeVertexNormal (const int vertex) |
| const NormalVector & | GetFaceNormal (const int face) const |
| const NormalVector & | GetVertexNormal (const int vertex) const |
| const NormalVector & | GetSurfaceNormal (const int id) const |
| const LibUtilities::PointsKeyVector | GetPointsKeys () const |
| Array< OneD, unsigned int > | GetEdgeInverseBoundaryMap (int eid) |
| Array< OneD, unsigned int > | GetFaceInverseBoundaryMap (int fid, StdRegions::Orientation faceOrient=eNoOrientation) |
| DNekMatSharedPtr | BuildInverseTransformationMatrix (const DNekScalMatSharedPtr &m_transformationmatrix) |
| void | PhysInterpToSimplexEquiSpaced (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| This function performs an interpolation from the physical space points provided at input into an array of equispaced points which are not the collapsed coordinate. So for a tetrahedron you will only get a tetrahedral number of values. | |
| void | GetSimplexEquiSpacedConnectivity (Array< OneD, int > &conn, bool standard=true) |
| This function provides the connectivity of local simplices (triangles or tets) to connect the equispaced data points provided by PhysInterpToSimplexEquiSpaced. | |
| template<class T > | |
| boost::shared_ptr< T > | as () |
 Public Member Functions inherited from Nektar::LocalRegions::Expansion3D Public Member Functions inherited from Nektar::LocalRegions::Expansion3D | |
| Expansion3D (SpatialDomains::Geometry3DSharedPtr pGeom) | |
| virtual | ~Expansion3D () |
| void | SetFaceExp (const int face, Expansion2DSharedPtr &f) |
| Expansion2DSharedPtr | GetFaceExp (const int face) |
| void | SetTraceToGeomOrientation (Array< OneD, NekDouble > &inout) |
| Align trace orientation with the geometry orientation. | |
| void | SetFaceToGeomOrientation (const int face, Array< OneD, NekDouble > &inout) |
| Align face orientation with the geometry orientation. | |
| void | AddHDGHelmholtzFaceTerms (const NekDouble tau, const int edge, Array< OneD, NekDouble > &facePhys, const StdRegions::VarCoeffMap &dirForcing, Array< OneD, NekDouble > &outarray) |
| void | AddNormTraceInt (const int dir, Array< OneD, ExpansionSharedPtr > &FaceExp, Array< OneD, Array< OneD, NekDouble > > &faceCoeffs, Array< OneD, NekDouble > &outarray) |
| void | AddNormTraceInt (const int dir, Array< OneD, const NekDouble > &inarray, Array< OneD, ExpansionSharedPtr > &FaceExp, Array< OneD, NekDouble > &outarray, const StdRegions::VarCoeffMap &varcoeffs) |
| void | AddFaceBoundaryInt (const int face, ExpansionSharedPtr &FaceExp, Array< OneD, NekDouble > &facePhys, Array< OneD, NekDouble > &outarray, const StdRegions::VarCoeffMap &varcoeffs=StdRegions::NullVarCoeffMap) |
| SpatialDomains::Geometry3DSharedPtr | GetGeom3D () const |
 Public Member Functions inherited from Nektar::LocalRegions::Expansion Public Member Functions inherited from Nektar::LocalRegions::Expansion | |
| Expansion (SpatialDomains::GeometrySharedPtr pGeom) | |
| Expansion (const Expansion &pSrc) | |
| virtual | ~Expansion () |
| DNekScalMatSharedPtr | GetLocMatrix (const LocalRegions::MatrixKey &mkey) |
| DNekScalMatSharedPtr | GetLocMatrix (const StdRegions::MatrixType mtype, const StdRegions::ConstFactorMap &factors=StdRegions::NullConstFactorMap, const StdRegions::VarCoeffMap &varcoeffs=StdRegions::NullVarCoeffMap) |
| SpatialDomains::GeometrySharedPtr | GetGeom () const |
| virtual const SpatialDomains::GeomFactorsSharedPtr & | v_GetMetricInfo () const |
| DNekMatSharedPtr | BuildTransformationMatrix (const DNekScalMatSharedPtr &r_bnd, const StdRegions::MatrixType matrixType) |
| DNekMatSharedPtr | BuildVertexMatrix (const DNekScalMatSharedPtr &r_bnd) |
| void | AddEdgeNormBoundaryInt (const int edge, const boost::shared_ptr< Expansion > &EdgeExp, const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, Array< OneD, NekDouble > &outarray) |
| void | AddEdgeNormBoundaryInt (const int edge, const boost::shared_ptr< Expansion > &EdgeExp, const Array< OneD, const NekDouble > &Fn, Array< OneD, NekDouble > &outarray) |
| void | AddFaceNormBoundaryInt (const int face, const boost::shared_ptr< Expansion > &FaceExp, const Array< OneD, const NekDouble > &Fn, Array< OneD, NekDouble > &outarray) |
| void | DGDeriv (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, ExpansionSharedPtr > &EdgeExp, Array< OneD, Array< OneD, NekDouble > > &coeffs, Array< OneD, NekDouble > &outarray) |
Protected Member Functions | |
| virtual NekDouble | v_Integral (const Array< OneD, const NekDouble > &inarray) |
| Integrate the physical point list inarray over prismatic region and return the value. | |
| virtual void | v_PhysDeriv (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_d0, Array< OneD, NekDouble > &out_d1, Array< OneD, NekDouble > &out_d2) |
| Calculate the derivative of the physical points. | |
| virtual void | v_FwdTrans (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| Forward transform from physical quadrature space stored in inarray and evaluate the expansion coefficients and store in (this)->m_coeffs. | |
| virtual void | v_IProductWRTBase (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| Calculate the inner product of inarray with respect to the basis B=base0*base1*base2 and put into outarray: | |
| virtual void | v_IProductWRTBase_SumFac (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | v_IProductWRTDerivBase (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
Calculates the inner product 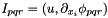 . . | |
| void | v_IProductWRTDerivBase_SumFac (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_GetCoord (const Array< OneD, const NekDouble > &Lcoords, Array< OneD, NekDouble > &coords) |
| Get the coordinates #coords at the local coordinates #Lcoords. | |
| virtual void | v_GetCoords (Array< OneD, NekDouble > &coords_1, Array< OneD, NekDouble > &coords_2, Array< OneD, NekDouble > &coords_3) |
| virtual NekDouble | v_StdPhysEvaluate (const Array< OneD, const NekDouble > &Lcoord, const Array< OneD, const NekDouble > &physvals) |
| virtual NekDouble | v_PhysEvaluate (const Array< OneD, const NekDouble > &coord, const Array< OneD, const NekDouble > &physvals) |
| This function evaluates the expansion at a single (arbitrary) point of the domain. | |
| virtual int | v_GetCoordim () |
| virtual void | v_ExtractDataToCoeffs (const NekDouble *data, const std::vector< unsigned int > &nummodes, const int mode_offset, NekDouble *coeffs) |
| Unpack data from input file assuming it comes from the same expansion type. | |
| virtual void | v_GetFacePhysVals (const int face, const StdRegions::StdExpansionSharedPtr &FaceExp, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, StdRegions::Orientation orient) |
| virtual void | v_GetTracePhysVals (const int face, const StdRegions::StdExpansionSharedPtr &FaceExp, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, StdRegions::Orientation orient) |
| Returns the physical values at the quadrature points of a face. | |
| void | v_ComputeFaceNormal (const int face) |
| virtual void | v_MassMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_LaplacianMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_LaplacianMatrixOp (const int k1, const int k2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_HelmholtzMatrixOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_GeneralMatrixOp_MatOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual DNekMatSharedPtr | v_GenMatrix (const StdRegions::StdMatrixKey &mkey) |
| virtual DNekMatSharedPtr | v_CreateStdMatrix (const StdRegions::StdMatrixKey &mkey) |
| virtual DNekScalMatSharedPtr | v_GetLocMatrix (const MatrixKey &mkey) |
| virtual DNekScalBlkMatSharedPtr | v_GetLocStaticCondMatrix (const MatrixKey &mkey) |
| void | v_DropLocStaticCondMatrix (const MatrixKey &mkey) |
| DNekScalMatSharedPtr | CreateMatrix (const MatrixKey &mkey) |
| DNekScalBlkMatSharedPtr | CreateStaticCondMatrix (const MatrixKey &mkey) |
| virtual void | v_GetSimplexEquiSpacedConnectivity (Array< OneD, int > &conn, bool standard=true) |
 Protected Member Functions inherited from Nektar::StdRegions::StdPrismExp Protected Member Functions inherited from Nektar::StdRegions::StdPrismExp | |
| virtual void | v_PhysDeriv (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| Calculate the derivative of the physical points in a given direction. | |
| virtual void | v_StdPhysDeriv (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &out_d0, Array< OneD, NekDouble > &out_d1, Array< OneD, NekDouble > &out_d2) |
| virtual void | v_StdPhysDeriv (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_BwdTrans (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_BwdTrans_SumFac (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_BwdTrans_SumFacKernel (const Array< OneD, const NekDouble > &base0, const Array< OneD, const NekDouble > &base1, const Array< OneD, const NekDouble > &base2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp, bool doCheckCollDir0, bool doCheckCollDir1, bool doCheckCollDir2) |
| virtual void | v_IProductWRTBase_MatOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_IProductWRTBase_SumFacKernel (const Array< OneD, const NekDouble > &base0, const Array< OneD, const NekDouble > &base1, const Array< OneD, const NekDouble > &base2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp, bool doCheckCollDir0, bool doCheckCollDir1, bool doCheckCollDir2) |
| virtual void | v_IProductWRTDerivBase_MatOp (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_LocCoordToLocCollapsed (const Array< OneD, const NekDouble > &xi, Array< OneD, NekDouble > &eta) |
| virtual void | v_FillMode (const int mode, Array< OneD, NekDouble > &outarray) |
| virtual int | v_GetNverts () const |
| virtual int | v_GetNedges () const |
| virtual int | v_GetNfaces () const |
| virtual LibUtilities::ShapeType | v_DetShapeType () const |
| Return Shape of region, using ShapeType enum list; i.e. prism. | |
| virtual int | v_NumBndryCoeffs () const |
| virtual int | v_NumDGBndryCoeffs () const |
| virtual int | v_GetEdgeNcoeffs (const int i) const |
| virtual int | v_GetTotalEdgeIntNcoeffs () const |
| virtual int | v_GetFaceNcoeffs (const int i) const |
| virtual int | v_GetFaceNumPoints (const int i) const |
| virtual LibUtilities::PointsKey | v_GetFacePointsKey (const int i, const int j) const |
| virtual const LibUtilities::BasisKey | v_DetFaceBasisKey (const int i, const int k) const |
| virtual int | v_GetFaceIntNcoeffs (const int i) const |
| virtual int | v_GetTotalFaceIntNcoeffs () const |
| virtual int | v_CalcNumberOfCoefficients (const std::vector< unsigned int > &nummodes, int &modes_offset) |
| virtual LibUtilities::BasisType | v_GetEdgeBasisType (const int i) const |
| virtual bool | v_IsBoundaryInteriorExpansion () |
| virtual void | v_GetFaceToElementMap (const int fid, const Orientation faceOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray, int nummodesA=-1, int nummodesB=-1) |
| virtual int | v_GetVertexMap (int localVertexId, bool useCoeffPacking=false) |
| virtual void | v_GetEdgeInteriorMap (const int eid, const Orientation edgeOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray) |
| virtual void | v_GetFaceInteriorMap (const int fid, const Orientation faceOrient, Array< OneD, unsigned int > &maparray, Array< OneD, int > &signarray) |
| virtual void | v_GetInteriorMap (Array< OneD, unsigned int > &outarray) |
| virtual void | v_GetBoundaryMap (Array< OneD, unsigned int > &outarray) |
| virtual void | v_MultiplyByStdQuadratureMetric (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | v_SVVLaplacianFilter (Array< OneD, NekDouble > &array, const StdMatrixKey &mkey) |
| virtual void | v_ReduceOrderCoeffs (int numMin, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
 Protected Member Functions inherited from Nektar::StdRegions::StdExpansion3D Protected Member Functions inherited from Nektar::StdRegions::StdExpansion3D | |
| virtual NekDouble | v_PhysEvaluate (const Array< OneD, DNekMatSharedPtr > &I, const Array< OneD, const NekDouble > &physvals) |
| virtual void | v_LaplacianMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_HelmholtzMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdRegions::StdMatrixKey &mkey) |
| virtual void | v_NegateFaceNormal (const int face) |
 Protected Member Functions inherited from Nektar::StdRegions::StdExpansion Protected Member Functions inherited from Nektar::StdRegions::StdExpansion | |
| DNekMatSharedPtr | CreateStdMatrix (const StdMatrixKey &mkey) |
| DNekBlkMatSharedPtr | CreateStdStaticCondMatrix (const StdMatrixKey &mkey) |
| Create the static condensation of a matrix when using a boundary interior decomposition. | |
| IndexMapValuesSharedPtr | CreateIndexMap (const IndexMapKey &ikey) |
| Create an IndexMap which contains mapping information linking any specific element shape with either its boundaries, edges, faces, verteces, etc. | |
| void | BwdTrans_MatOp (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | BwdTrans_SumFac (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | IProductWRTBase_SumFac (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | IProductWRTDerivBase_SumFac (const int dir, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| void | GeneralMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | MassMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LaplacianMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LaplacianMatrixOp_MatFree_Kernel (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp) |
| void | LaplacianMatrixOp_MatFree_GenericImpl (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LaplacianMatrixOp_MatFree (const int k1, const int k2, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | WeakDerivMatrixOp_MatFree (const int i, const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | WeakDirectionalDerivMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | MassLevelCurvatureMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | LinearAdvectionDiffusionReactionMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey, bool addDiffusionTerm=true) |
| void | HelmholtzMatrixOp_MatFree (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| void | HelmholtzMatrixOp_MatFree_GenericImpl (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, const StdMatrixKey &mkey) |
| virtual void | v_SetCoeffsToOrientation (StdRegions::Orientation dir, Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_SetCoeffsToOrientation (Array< OneD, NekDouble > &coeffs, StdRegions::Orientation dir) |
 Protected Member Functions inherited from Nektar::LocalRegions::Expansion3D Protected Member Functions inherited from Nektar::LocalRegions::Expansion3D | |
| virtual void | v_DGDeriv (const int dir, const Array< OneD, const NekDouble > &incoeffs, Array< OneD, ExpansionSharedPtr > &FaceExp, Array< OneD, Array< OneD, NekDouble > > &faceCoeffs, Array< OneD, NekDouble > &out_d) |
| Evaluate coefficients of weak deriviative in the direction dir given the input coefficicents incoeffs and the imposed boundary values in EdgeExp (which will have its phys space updated). | |
| virtual void | v_AddFaceNormBoundaryInt (const int face, const ExpansionSharedPtr &FaceExp, const Array< OneD, const NekDouble > &Fn, Array< OneD, NekDouble > &outarray) |
| virtual void | v_AddRobinMassMatrix (const int face, const Array< OneD, const NekDouble > &primCoeffs, DNekMatSharedPtr &inoutmat) |
| virtual StdRegions::Orientation | v_GetForient (int face) |
| virtual Array< OneD, unsigned int > | v_GetEdgeInverseBoundaryMap (int eid) |
| virtual Array< OneD, unsigned int > | v_GetFaceInverseBoundaryMap (int fid, StdRegions::Orientation faceOrient=StdRegions::eNoOrientation) |
| virtual DNekMatSharedPtr | v_BuildTransformationMatrix (const DNekScalMatSharedPtr &r_bnd, const StdRegions::MatrixType matrixType) |
| virtual DNekMatSharedPtr | v_BuildInverseTransformationMatrix (const DNekScalMatSharedPtr &m_transformationmatrix) |
Build inverse and inverse transposed transformation matrix: 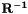 and and 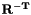 . . | |
| virtual DNekMatSharedPtr | v_BuildVertexMatrix (const DNekScalMatSharedPtr &r_bnd) |
 Protected Member Functions inherited from Nektar::LocalRegions::Expansion Protected Member Functions inherited from Nektar::LocalRegions::Expansion | |
| void | ComputeLaplacianMetric () |
| void | ComputeQuadratureMetric () |
| virtual void | v_MultiplyByQuadratureMetric (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray) |
| virtual void | v_ComputeLaplacianMetric () |
| virtual void | v_AddEdgeNormBoundaryInt (const int edge, const boost::shared_ptr< Expansion > &EdgeExp, const Array< OneD, const NekDouble > &Fx, const Array< OneD, const NekDouble > &Fy, Array< OneD, NekDouble > &outarray) |
| virtual void | v_AddEdgeNormBoundaryInt (const int edge, const boost::shared_ptr< Expansion > &EdgeExp, const Array< OneD, const NekDouble > &Fn, Array< OneD, NekDouble > &outarray) |
| virtual void | v_AddFaceNormBoundaryInt (const int face, const boost::shared_ptr< Expansion > &FaceExp, const Array< OneD, const NekDouble > &Fn, Array< OneD, NekDouble > &outarray) |
Private Member Functions | |
| virtual void | v_LaplacianMatrixOp_MatFree_Kernel (const Array< OneD, const NekDouble > &inarray, Array< OneD, NekDouble > &outarray, Array< OneD, NekDouble > &wsp) |
| Calculate the Laplacian multiplication in a matrix-free manner. | |
Additional Inherited Members | |
 Protected Attributes inherited from Nektar::StdRegions::StdExpansion3D Protected Attributes inherited from Nektar::StdRegions::StdExpansion3D | |
| std::map< int, NormalVector > | m_faceNormals |
| std::map< int, bool > | m_negatedNormals |
 Protected Attributes inherited from Nektar::LocalRegions::Expansion Protected Attributes inherited from Nektar::LocalRegions::Expansion | |
| SpatialDomains::GeometrySharedPtr | m_geom |
| SpatialDomains::GeomFactorsSharedPtr | m_metricinfo |
| MetricMap | m_metrics |
Definition at line 50 of file PrismExp.h.
| Nektar::LocalRegions::PrismExp::PrismExp | ( | const LibUtilities::BasisKey & | Ba, |
| const LibUtilities::BasisKey & | Bb, | ||
| const LibUtilities::BasisKey & | Bc, | ||
| const SpatialDomains::PrismGeomSharedPtr & | geom | ||
| ) |
Constructor using BasisKey class for quadrature points and order definition.
Definition at line 47 of file PrismExp.cpp.
| Nektar::LocalRegions::PrismExp::PrismExp | ( | const PrismExp & | T | ) |
Definition at line 69 of file PrismExp.cpp.
| Nektar::LocalRegions::PrismExp::~PrismExp | ( | ) |
Definition at line 80 of file PrismExp.cpp.
|
protected |
Definition at line 1231 of file PrismExp.cpp.
References ASSERTL2, Nektar::LocalRegions::Expansion::BuildTransformationMatrix(), Nektar::LocalRegions::Expansion::BuildVertexMatrix(), Nektar::StdRegions::StdMatrixKey::ConstFactorExists(), Nektar::StdRegions::StdExpansion::DetShapeType(), Nektar::SpatialDomains::eDeformed, Nektar::StdRegions::eFactorLambda, Nektar::StdRegions::eFactorSVVCutoffRatio, Nektar::StdRegions::eHelmholtz, Nektar::StdRegions::eHybridDGHelmBndLam, Nektar::StdRegions::eHybridDGHelmholtz, Nektar::StdRegions::eHybridDGLamToQ0, Nektar::StdRegions::eHybridDGLamToQ1, Nektar::StdRegions::eHybridDGLamToU, Nektar::StdRegions::eInvHybridDGHelmholtz, Nektar::StdRegions::eInvLaplacianWithUnityMean, Nektar::StdRegions::eInvMass, Nektar::StdRegions::eIProductWRTBase, Nektar::StdRegions::eLaplacian, Nektar::StdRegions::eLaplacian00, Nektar::StdRegions::eLaplacian01, Nektar::StdRegions::eLaplacian02, Nektar::StdRegions::eLaplacian11, Nektar::StdRegions::eLaplacian12, Nektar::StdRegions::eLaplacian22, Nektar::StdRegions::eMass, Nektar::SpatialDomains::eNoGeomType, Nektar::StdRegions::ePreconLinearSpace, Nektar::StdRegions::ePreconLinearSpaceMass, Nektar::StdRegions::ePreconR, Nektar::StdRegions::ePreconRMass, Nektar::StdRegions::ePreconRT, Nektar::StdRegions::ePreconRTMass, Nektar::StdRegions::eWeakDeriv0, Nektar::StdRegions::eWeakDeriv1, Nektar::StdRegions::eWeakDeriv2, Nektar::StdRegions::StdExpansion::GenMatrix(), Nektar::StdRegions::StdMatrixKey::GetConstFactor(), Nektar::StdRegions::StdMatrixKey::GetConstFactors(), Nektar::StdRegions::StdExpansion::GetLocStaticCondMatrix(), Nektar::StdRegions::StdMatrixKey::GetMatrixType(), Nektar::StdRegions::StdMatrixKey::GetNVarCoeff(), Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::StdRegions::StdMatrixKey::GetShapeType(), Nektar::StdRegions::StdExpansion::GetStdMatrix(), Nektar::StdRegions::StdMatrixKey::GetVarCoeffs(), m_matrixManager, Nektar::LocalRegions::Expansion::m_metricinfo, and Nektar::Transpose().
|
protected |
Definition at line 1548 of file PrismExp.cpp.
References ASSERTL2, Nektar::SpatialDomains::eDeformed, Nektar::StdRegions::eHelmholtz, Nektar::StdRegions::eLaplacian, Nektar::SpatialDomains::eNoGeomType, Nektar::StdRegions::StdExpansion::GetBoundaryMap(), Nektar::StdRegions::StdExpansion::GetInteriorMap(), Nektar::LocalRegions::Expansion::GetLocMatrix(), Nektar::StdRegions::StdMatrixKey::GetMatrixType(), Nektar::StdRegions::StdExpansion::GetStdStaticCondMatrix(), Nektar::LocalRegions::Expansion::m_metricinfo, Nektar::StdRegions::StdExpansion::m_ncoeffs, and Nektar::StdRegions::StdExpansion::NumBndryCoeffs().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 857 of file PrismExp.cpp.
References ASSERTL0, Nektar::StdRegions::StdExpansion::DetFaceBasisKey(), Nektar::SpatialDomains::eMovingRegular, Nektar::SpatialDomains::eRegular, Vmath::Fill(), Nektar::StdRegions::StdExpansion::GetCoordim(), Nektar::LocalRegions::Expansion::GetGeom(), Nektar::LibUtilities::BasisKey::GetNumPoints(), Nektar::LibUtilities::BasisKey::GetPointsKey(), Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::LibUtilities::Interp2D(), Nektar::StdRegions::StdExpansion3D::m_faceNormals, Vmath::Sdiv(), Vmath::Vmul(), Vmath::Vsqrt(), Vmath::Vvtvp(), and Vmath::Zero().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 1205 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::m_base.
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1226 of file PrismExp.cpp.
References Nektar::LibUtilities::NekManager< KeyType, ValueT, opLessCreator >::DeleteObject(), and m_staticCondMatrixManager.
|
protectedvirtual |
Unpack data from input file assuming it comes from the same expansion type.
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 501 of file PrismExp.cpp.
References ASSERTL0, ASSERTL1, Nektar::LibUtilities::eModified_A, Nektar::LibUtilities::eModified_B, Nektar::StdRegions::StdExpansion::GetBasisType(), Nektar::StdRegions::StdExpansion::m_base, Nektar::StdRegions::StdExpansion::m_ncoeffs, Vmath::Vcopy(), and Vmath::Zero().
|
protectedvirtual |
Forward transform from physical quadrature space stored in inarray and evaluate the expansion coefficients and store in (this)->m_coeffs.
Inputs:
Outputs:
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 215 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::DetShapeType(), Nektar::StdRegions::eInvMass, Nektar::eWrapper, Nektar::StdRegions::StdExpansion::GetNcoeffs(), Nektar::StdRegions::StdExpansion::m_base, m_matrixManager, Nektar::StdRegions::StdExpansion::m_ncoeffs, v_IProductWRTBase(), and Vmath::Vcopy().
|
protectedvirtual |
Definition at line 1155 of file PrismExp.cpp.
References Nektar::LocalRegions::Expansion::GetLocMatrix(), Nektar::StdRegions::StdExpansion::m_ncoeffs, and Vmath::Vcopy().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 1182 of file PrismExp.cpp.
References Nektar::StdRegions::eHybridDGHelmBndLam, Nektar::StdRegions::eHybridDGHelmholtz, Nektar::StdRegions::eHybridDGLamToQ0, Nektar::StdRegions::eHybridDGLamToQ1, Nektar::StdRegions::eHybridDGLamToQ2, Nektar::StdRegions::eHybridDGLamToU, Nektar::StdRegions::eInvLaplacianWithUnityMean, and Nektar::StdRegions::StdMatrixKey::GetMatrixType().
|
protectedvirtual |
Get the coordinates #coords at the local coordinates #Lcoords.
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 440 of file PrismExp.cpp.
References ASSERTL1, and Nektar::LocalRegions::Expansion::m_geom.
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion3D.
Definition at line 496 of file PrismExp.cpp.
References Nektar::LocalRegions::Expansion::m_geom.
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 458 of file PrismExp.cpp.
|
protectedvirtual |
Definition at line 572 of file PrismExp.cpp.
References ASSERTL0, Nektar::StdRegions::eDir1BwdDir1_Dir2BwdDir2, Nektar::StdRegions::eDir1BwdDir1_Dir2FwdDir2, Nektar::StdRegions::eDir1BwdDir2_Dir2BwdDir1, Nektar::StdRegions::eDir1BwdDir2_Dir2FwdDir1, Nektar::StdRegions::eDir1FwdDir1_Dir2BwdDir2, Nektar::StdRegions::eDir1FwdDir1_Dir2FwdDir2, Nektar::StdRegions::eDir1FwdDir2_Dir2BwdDir1, Nektar::StdRegions::eDir1FwdDir2_Dir2FwdDir1, Nektar::StdRegions::eNoOrientation, Nektar::StdRegions::StdExpansion::GetFaceNumPoints(), Nektar::StdRegions::StdExpansion::GetForient(), Nektar::LibUtilities::Interp2D(), Nektar::StdRegions::StdExpansion::m_base, and Vmath::Vcopy().
Referenced by v_GetTracePhysVals().
|
protectedvirtual |
Reimplemented from Nektar::LocalRegions::Expansion.
Definition at line 1216 of file PrismExp.cpp.
References m_matrixManager.
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1221 of file PrismExp.cpp.
References m_staticCondMatrixManager.
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1836 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::m_base, and Nektar::LocalRegions::Expansion::m_geom.
|
protectedvirtual |
Returns the physical values at the quadrature points of a face.
Definition at line 562 of file PrismExp.cpp.
References v_GetFacePhysVals().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1147 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion3D::v_HelmholtzMatrixOp_MatFree().
|
protectedvirtual |
Integrate the physical point list inarray over prismatic region and return the value.
Inputs:
Outputs:
![$\int^1_{-1}\int^1_{-1}\int^1_{-1} u(\bar \eta_1, \xi_2, \xi_3) J[i,j,k] d \bar \eta_1 d \xi_2 d \xi_3 $](form_228.png)
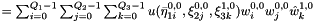
![$ inarray[i,j, k] = u(\bar \eta_{1i}^{0,0}, \xi_{2j}^{0,0},\xi_{3k}^{1,0}) $](form_230.png) ,
, 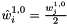
![$ J[i,j,k] $](form_219.png) is the Jacobian evaluated at the quadrature point.
is the Jacobian evaluated at the quadrature point. Reimplemented from Nektar::StdRegions::StdExpansion3D.
Definition at line 109 of file PrismExp.cpp.
References Nektar::SpatialDomains::eDeformed, Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::StdRegions::StdExpansion::m_base, Nektar::LocalRegions::Expansion::m_metricinfo, Vmath::Smul(), and Vmath::Vmul().
|
protectedvirtual |
Calculate the inner product of inarray with respect to the basis B=base0*base1*base2 and put into outarray:
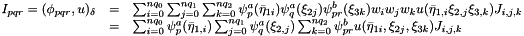
where
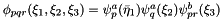
which can be implemented as
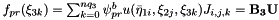
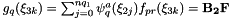
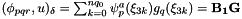
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 270 of file PrismExp.cpp.
References v_IProductWRTBase_SumFac().
Referenced by v_FwdTrans().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 277 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion3D::IProductWRTBase_SumFacKernel(), Nektar::StdRegions::StdExpansion::m_base, and Nektar::StdRegions::StdExpansion::MultiplyByQuadratureMetric().
Referenced by v_IProductWRTBase().
|
protectedvirtual |
Calculates the inner product 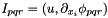 .
.
The derivative of the basis functions is performed using the chain rule in order to incorporate the geometric factors. Assuming that the basis functions are a tensor product 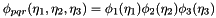 , this yields the result
, this yields the result
![\[ I_{pqr} = \sum_{j=1}^3 \left(u, \frac{\partial u}{\partial \eta_j} \frac{\partial \eta_j}{\partial x_i}\right) \]](form_233.png)
In the tetrahedral element, we must also incorporate a second set of geometric factors which incorporate the collapsed co-ordinate system, so that
![\[ \frac{\partial\eta_j}{\partial x_i} = \sum_{k=1}^3 \frac{\partial\eta_j}{\partial\xi_k}\frac{\partial\xi_k}{\partial x_i} \]](form_234.png)
These derivatives can be found on p152 of Sherwin & Karniadakis.
| dir | Direction in which to take the derivative. |
| inarray | The function 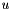 . . |
| outarray | Value of the inner product. |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 329 of file PrismExp.cpp.
References v_IProductWRTDerivBase_SumFac().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 337 of file PrismExp.cpp.
References Nektar::SpatialDomains::eDeformed, Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::StdRegions::StdExpansion3D::IProductWRTBase_SumFacKernel(), Nektar::StdRegions::StdExpansion::m_base, Nektar::LocalRegions::Expansion::m_metricinfo, Nektar::StdRegions::StdExpansion::m_ncoeffs, Nektar::StdRegions::StdExpansion::MultiplyByQuadratureMetric(), Vmath::Smul(), Vmath::Vadd(), and Vmath::Vmul().
Referenced by v_IProductWRTDerivBase().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1129 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::LaplacianMatrixOp_MatFree().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1137 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::LaplacianMatrixOp_MatFree().
|
privatevirtual |
Calculate the Laplacian multiplication in a matrix-free manner.
This function is the kernel of the Laplacian matrix-free operator, and is used in v_HelmholtzMatrixOp_MatFree to determine the effect of the Helmholtz operator in a similar fashion.
The majority of the calculation is precisely the same as in the hexahedral expansion; however the collapsed co-ordinate system must be taken into account when constructing the geometric factors. How this is done is detailed more exactly in the tetrahedral expansion. On entry to this function, the input #inarray must be in its backwards-transformed state (i.e. 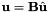 ). The output is in coefficient space.
). The output is in coefficient space.
Definition at line 1680 of file PrismExp.cpp.
References Nektar::SpatialDomains::eDeformed, Vmath::Fill(), Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::StdRegions::StdExpansion3D::IProductWRTBase_SumFacKernel(), Nektar::StdRegions::StdExpansion::m_base, Nektar::LocalRegions::Expansion::m_metricinfo, Nektar::StdRegions::StdExpansion::m_ncoeffs, Nektar::StdRegions::StdExpansion::MultiplyByQuadratureMetric(), Nektar::StdRegions::StdExpansion3D::PhysTensorDeriv(), Vmath::Svtsvtp(), Vmath::Svtvp(), Vmath::Vadd(), Vmath::Vvtvp(), and Vmath::Vvtvvtp().
|
protectedvirtual |
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 1121 of file PrismExp.cpp.
References Nektar::StdRegions::StdExpansion::MassMatrixOp_MatFree().
|
protectedvirtual |
Calculate the derivative of the physical points.
The derivative is evaluated at the nodal physical points. Derivatives with respect to the local Cartesian coordinates.
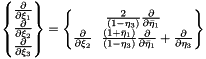
Reimplemented from Nektar::StdRegions::StdPrismExp.
Definition at line 135 of file PrismExp.cpp.
References Nektar::SpatialDomains::eDeformed, Nektar::StdRegions::StdExpansion::GetPointsKeys(), Nektar::StdRegions::StdExpansion::GetTotPoints(), Nektar::LocalRegions::Expansion::m_metricinfo, Vmath::Smul(), Vmath::Vmul(), and Vmath::Vvtvp().
|
protectedvirtual |
This function evaluates the expansion at a single (arbitrary) point of the domain.
Based on the value of the expansion at the quadrature points, this function calculates the value of the expansion at an arbitrary single points (with coordinates 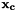 given by the pointer coords). This operation, equivalent to
given by the pointer coords). This operation, equivalent to
![\[ u(\mathbf{x_c}) = \sum_p \phi_p(\mathbf{x_c}) \hat{u}_p \]](form_127.png)
is evaluated using Lagrangian interpolants through the quadrature points:
![\[ u(\mathbf{x_c}) = \sum_p h_p(\mathbf{x_c}) u_p\]](form_128.png)
This function requires that the physical value array  (implemented as the attribute #phys) is set.
(implemented as the attribute #phys) is set.
| coords | the coordinates of the single point |
Reimplemented from Nektar::StdRegions::StdExpansion3D.
Definition at line 479 of file PrismExp.cpp.
References ASSERTL0, and Nektar::LocalRegions::Expansion::m_geom.
Referenced by v_StdPhysEvaluate().
|
protectedvirtual |
Given the local cartesian coordinate Lcoord evaluate the value of physvals at this point by calling through to the StdExpansion method
Reimplemented from Nektar::StdRegions::StdExpansion.
Definition at line 471 of file PrismExp.cpp.
References v_PhysEvaluate().
|
private |
Definition at line 206 of file PrismExp.h.
Referenced by CreateMatrix(), v_FwdTrans(), and v_GetLocMatrix().
|
private |
Definition at line 208 of file PrismExp.h.
Referenced by v_DropLocStaticCondMatrix(), and v_GetLocStaticCondMatrix().
 1.8.1.2
1.8.1.2